We are currently wondering why the 1550 nm light is not co-resonant with the 775 nm light, used to lock the GQuEST Output Filter Cavities, over time. Some ideas are the AOM frequency drifts (unlikely), but a potential culperate is differential length changes of the cavity due to thermooptic effects (thermal expansion and thermo-refraction). For light that hits the surface of the coating, Evans (2008) (Eq. 3) gives the sensitivity of the sensed position of the mirror \Delta T to temperature fluctuations T as

\[ \frac{\partial \Delta z}{\partial T} = \bar{\alpha}_c h_c - \bar{\beta}\lambda \]

Where \bar{\alpha}_c is the effective coefficient of thermal expansion, h_c is the coating thickness, \bar{\beta} is the effective thermo-refractive coefficient, and \lambda is the wavelength. This expresion doesn't work for the 775 nm light since it is below a layer of 1550 nm coating. Assume a fraction (\gamma) of the coating is for 1550 nm light and (1-\gamma) of the coating is for 775 nm light. \gamma \approx 0.5, although the 775 nm light coating is probably thinner since the fractional wavelength stacks are thinner. This analysis might also not be exact for the mirrors since none of them are HR for both wavelengths.

\[ \frac{\partial \Delta z_{775~\text{nm}}}{\partial T} = (1-\gamma)\bar{\alpha}_c h_c - \gamma\bar{\alpha}_c h_c(n-1) - \bar{\beta}( \gamma h_c+\lambda) \]

Where n is the index of refraction of 775 nm light in the 1550 nm coating. There is a minus sign in front of it because a higher index causes more phase to be accumulated than in air.

Now consider the differential sensitivity of the sensed position of the mirror, assuming the thermorefractive coefficient is very roughly the same for both wavelengths.

\[ \Delta \frac{\partial \Delta z}{\partial T} \equiv \frac{\partial \Delta z_{1550~\text{nm}}}{\partial T} -  \frac{\partial \Delta z_{775~\text{nm}}}{\partial T}\]

\[ \Delta \frac{\partial \Delta z}{\partial T} = \gamma h_c (\bar{\alpha}_c n + \bar{\beta}) \]

Plugging in numbers from our GQuEST Paper Table II, 

\[ \Delta \frac{\partial \Delta z}{\partial T} \approx 2 \cdot 10^{-10}~\text{m/K} \]

I'm not sure if ~1/1000 of a wavelength can explain the drift in the co-resonance over time.

-There is no beat note if the tank circuit on the 1550 EOM is removed.

-If the tank circuit for the 775 is removed the same 120 Hz oscillations are observed. The PDH error amplitude is also very small, as expected.

-I am going to remake a tank circuit and plan to operate OFC1 at 70 MHz (~20 MHz difference, should be filtered out by the low pass in the laser lock box).

Torrey asks here whether the Output Filter Cavity Offset Frequency can fluctuate from the Nominal Value of 17.6 MHz. If we assume 4 cavities have the same single cavity bandwidth of \Delta epsilon_1 and the same frequency offset, then the integrated transmission is around \Delta epsilon_1 / 2 (see section A.9 in our GQuEST paper). If we assume that 3 cavities have the same frequency offset and assume an allowable additional 5% signal transmission loss due to this mechanism, then the frequency can shift 16% of the bandwidth away from the nominal offset.

I derived this by integrating 4 Lorentizans, once with all 4 with the same offset and once with one with a deviated offset. I then figure out the deviation required so that the transmitted bandwidth is 95% of the no deviation case. For our bandwidth of 42 kHz, this is a value of 6.6 kHz.

If this single cavity had a fluctuating offset, then the RMS discrepancy between this cavity could probably be \sqrt{2} larger because it spends time near no offset deviation. I am assuming there are no dynamic effects of changing the offset when the round trip time in the cavity times the frequency fluctuation is much less than 1, which is the case becuase t_cav*f_bandwidth = 1/2pi.

If I make an assumption that at any given time the 4 cavities are randomly distributed, let's say "equally spaced" on a gaussian with z-scores of -0.84, -0.25, 0.25, and 0.84 (the integral from one value to the next, including +/- infinity, is 0.2), then the 1 sigma error for 95% transmission is 11% of the bandwidth away from the nominal offset, or 4.6 kHz. This is only a rough estimate and more sophisticated methods should be used if we want a more exact answer, but a good sanity check is that it is below the 1 cavity answer.

For OFC2, the laser lock quality seems poor. This and this show the 775 error signal used to lock the cavity in red, and blue shows the TRANS PD for 1550. I am not sure how to quantify a "good" lock in these cavities. For any diagnostics, this is a poor lock quality as the total 1550 transmission is fluctuating by alot. But in GQuest operation mode we will have it detuned by 17.6 MHz, thus supressing 1550 light. If it jitters around 17.6 MHz, still suppressing the carrier and allowing signal photons through, is this still an issue? We cannot suppress those fluctuations further, even though increasing the UGF of the loop squashes these fluctuations. At a certain point, something rings up (in the laser dc modulation port? elsewhere?) and doesn't allow further supression.

I am attempting to look at this issue on OFC1. It is largely the same. One major problem I diagnosed is having both EOM phase modulations on in one cavity at the same time. The clock sync that we have between the 4 mokus seems to not be sufficient. The signal is fluctuating multiple times a second. One moment and the next. This may not seem like a big deal but since the two wavelengths are tracking eachother, having a 1mV difference in the average level of the error point has the effect of detuning the 775 light away from the 1550 peak about a FWHM distance (read: when the average error point is 0, they are coaligned, when the average is lower, the 1550 TRANS light jumps to about half the value). Turning off the 1550 EOM signal gets rid of it. This is when the two EOMs are at the same frequency. When they aren't there is a large beat note in the error signal.

I would like to do some analysis on the filter cavities but am getting hung up on the fact the 1550 PDH error amplitude is very low. I've double checked the EOM frequency, inductor tuning in the tank circuit, and phase of the PDH loop. All seem fine. I believe it's somehow related to alignment, the REFL dips on the OFC1 1550 path are the lowest out of the 3 REFL PDs I have installed so far ( OFC1 1550, OFC1 775, OFC2 775). Below are screenshots of the error amplitude. If anyone has any other ideas to improve this please let me know. It wasn't this small in the past.

OFC1 1550

OFC1 775

OFC2 775

I scanned the laser on OFC2 for the first time since yesterday. There were higher order mode peaks on the 775 transmission PD at values higher than previously observed. This is most likely due to the earthquake that occured very near campus. I touched up the input alignment and now have almost no higher order modes seen on the TRANS PD.

[Erin, Daniel] 

Last Friday, Daniel and I pressed the end mirror mount to try to get different wavefronts from the mirror. The three 'shapes' the mirror is able to produce are a kind of X, an O, and a + (see the third row of Zernike polynomials below for what they look like). We just tried to produce X and the O, since they are the two most straight foward. 

We first took a starting measurement of the mirror with the Shack-Hartmann sensor. This is just to make sure that pressing the mirror still allows us to return to its original state after (it should, this was just a precaution). 

To press in the X, the four screws in the back (side facing us when you look at the table) were engaged (turned until they made contact with the mirror), and two screws in the front were. One suggestion was to place a multimeter on the mirror and the screw so that we could determine exaclty when the screw makes contact with the mirror. Unfortunately, since the entire mount is metal, this will cause the multimeter to short, so this probably won't work. Instead we found it was effective to lightly twist the screwdriver, holding it closer to the screw to apply less torque. After the screws were engaged, we turned them about 45 degrees, since 1mD of curvature is supposed to correspond to 5 degrees of turning (according to the COMSOL simulation. Then we took an image with the Shack-Hartmann sensor. 

After that, we disengaged the screws and took another image. Then, in order to press the O, we enaged 4 screws both in the front and in the back and turned each of the screws 360 degrees. 

Then we disengaged and took a final image. One challenge that might come up is adjusting the modes of the mirror without moving it relative to the sensor or changing the tilt. We found we had to adjust the mirror after pressing it cause it could easily get bumped. Analyzing the data is still in progress, I have a way to reconstruct the wavefront, calculate the radius of curvature, and the next step is to find a way to overlay the engaged and disengaged images to see how the Shack-Hartmann data changes between them. 

[Briana, Ian]

Wiggles in probe scan:

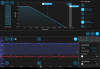
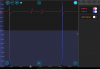
The wiggles tend to be about 0.4 mV in amplitude and a frequency of 4.6 Hz, as seen here. When we are not applying any modulation frequency or temperature scan, we see some variation in power because of the issue with the polarization-maintaining fiber, as seen here. On shorter timescales (zoom in a bunch), there is an additional ~60Hz oscillation, which is apparent whether you're scanning temperature or not. I think the wiggles have been there all along but were just less obvious when I zoomed into the main peak or zoomed out on the power scale. 

My best guess right now is that the power fluctuations are interfering with the scan features in the scanning signal. 

Things that don't affect the squiggles: decreasing the current, moving the fiber, scanning manually. Changing the scan frequency by a factor of two also decreases the frequency of this wiggle by approxiately a factor of 2 as well. Amplitude remains relatively unchanged. mod_200_20240812_174825_Screenshot.png

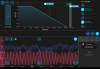
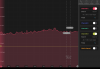
However, by tuning the modulation frequency/phase, you can suppress some of the oscillations in the error signal and also reach zero offset. If you choose a high modulation frequency, as seen here, the wiggles become much more obvious in the error signal. Based on my zooming, the wiggles' effect on the actual asymmetric error signal don't seem to be that significant, although it's still not ideal for them to exist. 

Why is the error signal offset?

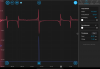
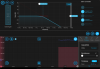
The error signal, when locked, is not at 0 Volts, it is some offset away as seen in this image (purple is error signal, red is photodetector): offsetafterlock_20240812_182448_Screenshot.png. I think what's going on is because the zero-crossing point is offset from the minimum of the peak, the systems locks (see errorsignaloffset.png) to where the error signal intersects the absorption dip minimum. At error signal = 0, the temperature is detuned from the absorption, so the error signal needs to be at an offset. 

Other:

Optimized PID controls of the vapor cell, which you can do automatically by going into Menu -> Ch 1 PID controller -> Auto-tune. Now, the temperature varies at most by 0.05 Celsius in comparison to the 0.5 Celsius oscillations before. This did not fix the wiggles. 

I locked with just the probe at an integrator unity gain frequency of 7.029 Hz and an integrator saturation level of 6.0 dB. After being enlightened about the matplotlib PSD function, this may be the ideal way to get the power spectrum since the Moku is limited at lower frequencies. We took data with a sample acquitsition frequency of 5 kSa/sec for 20 seconds when the laser was locked and not locked. Then, we get the PSD with the following parameters which follows a good averaging method (Welch method): detrend = linear to remove any linear shape in the segments of the power spectrum, Hanning window which is most widely used for making the signal as periodic as possible, NFFT = 256 for standard number of frequency points in the FFT. 

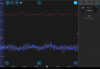
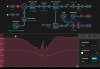
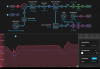
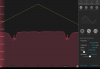
Lee suggested bit noise from the Moku may explain the previous noise spectra. We want to minimize quantization steps in the digitization process (converting the voltage reading to a digital value), so to do this we want to increase the analog signal as much as possible. Increasing the gain on the signal would accomplish this even though the gain amplifies other signal noise. I have not figure out how to increase the gain of the error signal because it might not be possible to increase the gain at a probe point in the laser lock box in the Moku. You could add in the lock-in amplifier but it's a hassle for tuning parameters. Amplifying just the input signal has not proven successful for increasing the error signal. For now, I've taken data with DC at different impedances, which should give an "estimate" for the different gains. The PSDs are shown here: 50_Ohm_impedance_comparison.png, 1_MOhm_impedance_comparison.png. At a higher "gain" (in this case, 1 MOhm impedance means the divider effect is closer to 1 compared to the 50 Ohm impedence which would divide out the signal by some factor less than 1), we would expect more noise so this matches well with the 1 MOhm vs. 50 Ohm PSD comparison. There seems to be no distinction between the locked and unlocked case at either impedance and artifacts in the 50 Ohm one that are not present in the 1 MOhm, not sure why but will retake data tomorrow to make sure it was not some silly mistake. 

I moved the KJL Boxes for GQuEST to B111B. I didn't move other components like Thorlabs mounts or screws.

The boxes on the shelves and/or on other boxes are fairly light.

We have detached the light fixture that was blocking the movement of the east moveable cleanroom enclosure in B111B.

The wires that suspend these lights are attached to metal brackets that are screwed into the light casing. Once the screws are removed, the brackets can be removed by prying them out of the plastic casing with a screwdriver. This releases the light, apart from the electrical connection to the ceiling. The electrical wire from the ceiling connects to the wiring inside the light with wire nuts, and cannot easily be detached.  

We released the light fixture from its suspension wires, and rested it on top of the moveable cleanroom. We then moved the cleanroom to adjoin the other one. The electrical wire is long enough to allow some movement of the cleanroom with the light resting on top of it, but we should not try moving the cleanroom more than ~30 cm or so without supporting the light.

Aligned pump. It is easier to align the probe before the pump. Upon scanning, we get the following signals (red is the photodetector signal, blue is the error signal): errsignal_bothpeaks_20240810_132203_Screenshot.png. You can see that the main dip has a peak in the middle (expected by the pump) although it is accompanied by some other weird peaks. The background while scanning is extremely noisy, which could  contribute to these peaks/oscillations in the dip. Additionally, the smaller transition (on the right) now has some extruding peak. A clearer view of the main peak is shown here where we are scanning across temperature: pumpprobe_20240810_130408_Screenshot.png. 

The error signal does not appear to be asymmetric. However, If you change the phase and modulation frequency, it begins to look a bit different and more asymmetric (contrast between differrsignal.png and errsignal_20240810_132122_Screenshot.png). 

One issue is the bumps when scanning temperature, which are apparent in the probe and pump. They are especially obvious when we just measure the probe (blocking the pump), as shown in probe_20240810_125659_Screenshot.png). These tiny wiggles are going to appear in the error signal so we need to find a way to remove them. 

Setup: IMG_2425.jpg

[Briana, Ian, Torrey]

In my previous post(s), I was taking the noise spectrum of the photodetector signal. I should be taking the noise spectrum of the error signal because 1). the error signal tells you how much the laser is actually drifted and 2). the photodetector signal includes additional effects from the EOM modulation.

Controller

The reason why there is no distinction of noise between the unlocked/locked case at high frequencies is that we are controlling the laser frequency by changing temperature, which is a slow actuator. If we can apply the fast controller to an EOM, we can adjust the frequency of the laser through this phase modulation, adding a fast actuator to our slow (temperature) actuator. Also, there is tradeoff with the gain of the controller. If the gain is too high, your system will be stabilized but there will be a lot of noise. If the gain is too low, then your controller won't be doing anything. Essentially, the gain parameters need to be just right so that the noise spectrum doesn't become amplified. Although useful for stabilization, we are not using the derivative control at all in the slow PID controller because this is a rapidly changing system, so we want the controller to be responsive to those changes. 

Unfortunate noise spectra

At low frequencies, the noise from the locked laser is lower than that from the not locked. At higher frequencies, the locked laser is noisier than the not locked laser. However, I would expect that because of the higher RMS and standard deviation of the error signal when unlocked, the noise should be higher for the unlocked, so it is not clear to me why it is behaving like this. This has been the case for different controllers at unity gain frequencies from 3 Hz to a few hundred Hz. An example of this is using locked5 data (see Nextcloud Users/briana/8_9_2024: LockedUnlockedNoiseSpectra.png with the following controller.

I took data for locked/not locked with a ~3 Hz integrator unity gain frequency and low ~2 dB integrator saturation level (data in NextCloud Users/briana/8_10_2024/noise spectrum probe test). The phasemeter and spectrum analyzer PSD disagree in which situation has the highest noise, although they are in different units. I have not found how the phasemeter converts to Hz/sqrt(Hz), so this could be a reason for the diagreement. Also, I am now again doubtful of the expected order of magnitude of the frequency noise spectrum after looking at the phasemeter ASD. 

Should we use the phasemeter? According to Liquid Instruments, the phasemeter measures frequencies around some acquisition frequency and uses a feedback loop to determine the phase of the input. The signal passes through a mixer and lowpass filter but the signal is mixed with some oscillator. This feedback loop tries to minimize the difference between the input and output frequency, which allows it to determine the frequency/phase. Another oscillator allows it to measure the amplitude. The phasemeter operates on a phase-locked loop, meaning you try to keep the phase of the output constant compared to the phase of the input. Because of this, you need to ensure a phase lock can occur (steady input signal needed), which is more difficult at low frequencies. So, this may not be the optimal instrument for measuring this signal since it relies on a lock and our signal only is affected at low frequencies.

I am going to do a more systematic test of the controller parameters (different gains, unity gain frequencies). I was previously just playing around with values but not documenting the controller well. I will also start taking unlocked laser noise spectra far from resonance to see if the way I'm taking data is causing this phenomenon.

Potential reasons for why locked noise spectrum has more noise?

• Controller issue (feedback gain is too high). I wonder if the shifting polarization of the laser is an issue because that introduces intensity noise, which may start dominating even more with the feedback.

• As Torrey pointed out, it could be that there is more noise with the lock because of shot noise (at the absorption dip, you have less photons so shot noise should increase). 

• Asymmetry of the error signal shouldn't matter because we just care about the linear region. Can the offset of the error signal from zero be an issue? Changing the modulation frequency (not phase shift) shifts the error signal up to avoid offset at the cost of error signal maximization. 

• Is it possible for the zero-crossing point to shift? Ian suggested that if the zero crossing point shifts, the frequency discrimination might be lower, especially if the zero crossing point reaches the ends of the linear region. The system is now not sensitive (the error signal is smaller for larger frequency deviation) so it is less good at reducing noise. 

• Mode hopping of laser. Probably not because this would cause sharp changes in the locking/photodetector signal, which is not apparent in our data (article by rp-photonics.com). 

Other

From Thorlabs specs, the EOM should actually be operating between DC and 100 MHz. Somehow, operating at 120 Hz gave the most symmetric and maximized error signal (400 microvolts amplitude), but it is clearly not built to operate at this level. Anyways, the maximized error signal is now at an EOM driving frequency of 48 MHz (200 microvolts in the positive y direction, 150 in the negative y direction).

We got the isomet amplifiers. These are higher efficiency and can be modulated, so you can make intensity stabilization and such. I put one in a chassis with power supply. You could put 2 - 4 of them as a package. Use a switches and headers to allow easily swapping amps or supplies.

The isomet amps have some LIGO docs up at https://dcc.ligo.org/LIGO-T1900049

This chassis setup can/should be used to package the minicircuits amps as well.

I created a finesse model a single cavity then I added all i = m + n higher order modes (HOMs). I then multiplied four of these cavities with varying length and crusher ROCs together to create a single plot of the higher mode leakage through the higher cavities. The HOMs  height was chosen to match the cavity scans that torrey did on OFC1 which roughly showed that the i=1 mode was 1/10th the power of the i=0 modes and the i=2 modes were 1/100th of the i=0 modes. With these HOM amplitudes and the four cavities multiplied together we have a good model of the HOM leakage in the output filter cavities shown in the attachment: multi_cavity_modes.pdf. The model goes up to n+m = 10 HOMs. Since HOMs contribute less and less as they go up in n+m it is not likely that higher HOMs would change these results significantly. The code for this model is stored in the gquest modeling git. The plot shows the bandpass for the signal at 17.6 MHz and the suppression at the carrier frequency of 0 Hz is a little less than 10^24 in power suppression. The only major improvement to this method is to normalize the power correctly. Currently when you add a HOM it decreases the power in the i=0 mode. so as I add more modes the bandpass section stops having a gain of 1. I have temporarily solved that by increasing the power input from 1 W to 1.3 W which seems to normalize correctly. Fixing this in the future could help reduce uncertainty in this plot. Another difference between this and the real experiment is that I have assumed that the HOM distribution and relative power exiting the IFO will match the laser that we are shining into the OFCs for testing. This should still give us a better understanding of of the performance of the cavities and will give us a comparison when we are doing our final cavity scans of all four cavities. We can also use this to change how the four filter cavities are spaced and their ROCs.

Previously, Torrey had to divide out the controller from the control signal to get the error signal. You can avoid all that if you just use the built-in math channel on the laser lock box and set it to take an FFT at a probe point. 

[Alex, Daniel]

I loaned out our RFSoc to the Hutzler Lab. The point of contact is Harish Ramachandran. Also in the loan was the included power cord and some SMA connectors.